Bull. Austral. Math. Soc. 72(2) pp.291--298, 2005.

Bounded vector measures on effect algebras

Hong Taek Hwang

Longlu Li

Hunnam Kim

Received: 4th May, 2005

This paper was supported by Kumoh National Institute of Technology.

Abstract

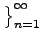Let (L, $\bot$ , $\oplus$ , 0, 1) be an effect algebra and X a locally convex space with dual X. A function $\mu$ : L $\rightarrow$ X is called a measure if $\mu$ (a $\oplus$ b) = $\mu$ (a) + $\mu$ (b) whenever a $\bot$ b in L and it is bounded if $\bigl \{$ $\mu$ (a_n) $\bigr \}_{{n=1}}^{{\infty }}$ is bounded for each orthogonal sequence {a_n} in L. We establish five useful conditions that are equivalent to boundedness for vector measures on effect algebras.

Click to download PDF of this article (free access until July 2006)

or get the no-frills version

[an error occurred while processing this directive]

(Metadata: XML, RSS, BibTeX)

MathSciNet: MR2183410

Z'blatt-MATH: 02246391

References

S.P. Gudder;
Quantum probability (Academic Press, Boston, 1989). MR958911
E.D. Habil;
Brooks-Jewett and Nikodym convergence theorems for orthoalgebras that have the weak subsequential interpolation property,
Internat. J. Theoret. Phys. 34 (1994), pp. 465--491. MR1330347
R.L. Li, R.C. Cui and M.H. Zhao;
Invariants on all admissible polar topologies,
Chinese Ann. Math. Ser. A 19 (1998), pp. 289--294. MR1641098
R. Li and S.M. Kang;
Characterizations of bounded vector measures,
Bull. Korean Math. Soc. 37 (2000), pp. 209--215. MR1757488
R.L. Li and Q.Y. Bu;
Locally convex spaces containing no copy of c₀,
J. Math. Anal. Appl. 172 (1993), pp. 205--211. MR1199505
F.G Mazario;
Convergence theorems for topological group valued measures en effect algebras,
Bull. Austral. Math. Soc. 64 (2001), pp. 213--231. MR1860059
C. Swartz and C. Stuart;
Orlicz-Pettis theorems for multiplier convergent series,
Z. Anal. Anwendungen 17 (1998), pp. 805--811. MR1669893
A. Wilansky;
Modern methods in topological vector spaces (McGraw-Hill, New York, 1978). MR518316

ISSN 0004-9727

Bulletin of the Australian Mathematical Society