J. Aust. Math. Soc.  82 (2007), 133-147
On values taken by the largest prime factor of shifted primes

William D. Banks
  Department of Mathematics
  University of Missouri
  Columbia, MO 65211
  USA
  bbanks@math.missouri.edu
and
Igor E. Shparlinski
  Department of Computing
  Macquarie University
  Sydney, NSW 2109
  Australia
  igor@ics.mq.edu.au


Abstract
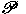Let $\mathcal{P}$ denote the set of prime numbers, and let $P(n)$ denote the largest prime factor of an integer $n>1$. We show that, for every real number $32/17<\eta<(4+3\sqrt{2})/4$, there exists a constant $c(\eta)>1$ such that for every integer $a\ne 0$, the set
 
\[ \bigl\{p\in\mathcal{P}:p=P(q-a)\text{ for some prime }q \text{ with }p^\eta<q<c(\eta)\,p^\eta\bigr\} \]
 
has relative asymptotic density one in the set of all prime numbers. Moreover, in the range $2\le\eta<(4+3\sqrt{2})/4$, one can take $c(\eta)=1+\varepsilon$ for any fixed $\varepsilon>0$. In particular, our results imply that for every real number $0.486\le\vartheta\le 0.531$, the relation $P(q-a)\asymp q^{\vartheta}$ holds for infinitely many primes $q$. We use this result to derive a lower bound on the number of distinct prime divisors of the value of the Carmichael function taken on a product of shifted primes. Finally, we study iterates of the map $q\mapsto P(q-a)$ for $a>0$, and show that for infinitely many primes $q$, this map can be iterated at least $(\log \log q)^{1+o(1)}$ times before it terminates.
Download the article in PDF format (size 155 Kb)
Australian Mathematical Publishing Association Inc. ©  Australian MS