J. Aust. Math. Soc.
75 (2003), 9-21
|
Multiplicities of higher Lie characters
|
|
|
Abstract
|
The higher Lie characters of the symmetric group
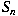 arise from the Poincaré-Birkhoff-Witt
basis of the free associative algebra. They are
indexed by the partitions of arise from the Poincaré-Birkhoff-Witt
basis of the free associative algebra. They are
indexed by the partitions of 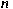 and sum up to the regular character of and sum up to the regular character of
 . A combinatorial description of the
multiplicities of their irreducible components is
given. As a special case the
Kraskiewicz-Weyman result on the
multiplicities of the classical Lie character is
obtained. . A combinatorial description of the
multiplicities of their irreducible components is
given. As a special case the
Kraskiewicz-Weyman result on the
multiplicities of the classical Lie character is
obtained.
|
Download the article in PDF format (size 110 Kb)
|
|
|
|