In this paper, first, we study the existence of
the positive solutions of the nonlinear elliptic
equations in unbounded domains. The existence is
affected by the properties of the geometry and
the topology of the domain. We assert that if
there exists a
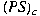 -sequence with -sequence with
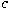 belonging to a suitable interval depending by
the equation, then a ground state solution and a
positive higher energy solution exist, too. Next,
we study the upper half strip with a hole. In
this case, the ground state solution does not
exist, however there exists at least a positive
higher energy solution. belonging to a suitable interval depending by
the equation, then a ground state solution and a
positive higher energy solution exist, too. Next,
we study the upper half strip with a hole. In
this case, the ground state solution does not
exist, however there exists at least a positive
higher energy solution.
|