J. Aust. Math. Soc.
77 (2004), 29-46
|
Wavelet decomposition of Calderón-Zygmund operators on function spaces
|
Ka-Sing Lau
Department of Mathematics
The Chinese University of Hong Kong
Shatin, NT
Hong Kong
kslau@math.cuhk.edu.hk
|
|
|
Lixin Yan
Department of Mathematics
Zhongshan University
Guangzhou, 510275
P. R. China
lixin@ics.mq.edu.au
|
|
|
Abstract
|
We make use of the Beylkin-Coifman-Rokhlin
wavelet decomposition algorithm on the
Calderón-Zygmund kernel to obtain some
fine estimates on the operator and prove
the 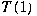 theorem on Besov and Triebel-Lizorkin spaces.
This extends previous results of Frazier et al., and Han and Hofmann.
|
Download the article in PDF format (size 149 Kb)
|
|
|
|