J. Aust. Math. Soc.
81 (2006), 279-295
|
Automatic continuity for Banach algebras with finite-dimensional radical
|
|
|
Abstract
|
The paper \cite{dalesloy1997} proved a necessary
algebraic condition for a Banach algebra
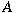 with finite-dimensional radical with finite-dimensional radical
 to have a unique complete (algebra) norm, and
conjectured that this condition is also
sufficient. We extend the above theorem. The
conjecture is confirmed in the case where to have a unique complete (algebra) norm, and
conjectured that this condition is also
sufficient. We extend the above theorem. The
conjecture is confirmed in the case where
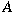 is separable and is separable and
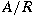 is commutative, but is shown to fail in general.
Similar questions for derivations are discussed. is commutative, but is shown to fail in general.
Similar questions for derivations are discussed.
|
Download the article in PDF format (size 128 Kb)
|
|
|
Australian Mathematical Publishing Association Inc.
|
©
Australian MS
|
|